Relativity
The Einstein's theory of relativity is based on the principle that the
physical laws are the same to every freely moving observer, whatever his
velocity is. That means that we all shall measure the same light speed, no matter
the direction to which we move and how fast we do it. According to this theory,
nothing can be faster than light (299 792,5 km/second), as it is demonstrated
next.
Einstein proved that:
|
______ ______E = |
__Mc2__ |
|
(1-s2/c2)1/2 |
E = energy
M = mass
c = speed of light
s = speed of the moving
object
When s is close to 0 (as it is for the velocities reached by human
artifacts), then we get:
E = Mc2
On the other hand, P can symbolize the linear momentum, or the total quantity
of movement, and now we get:
|
____________
P = |
__Ms___ |
|
(1-s2/c2)1/2 |
At minimal velocities we obtain:
P = Ms
The first equation of P tells us that when s becomes close to c (the speed
of light), then P approaches the infinite. So, s can never overtake c.
The Rhythm of Time
We have already seen that the speed of light is the same for an observer
who is static regarding to a reference vector and to an observer who is moving in
regard to that reference vector. If we suppose that the static observer watches
the light moving 10 metres and that the moving observer advances in the
direction of the photon (light particle) at 1/5 of its speed, then the latter
will advance 2 metres and will see the photon moving away only 10 - 2 = 8
metres. So, the light will have traveled different distances for each one of
them. As the speed of light is constant and as Velocity (speed) = Distance /
Time, then:
Time(divergent for the 2 observers) = Distance(divergent) /
Velocity(equal)
We conclude that the time flows at a different rate for a moving
observer and for a stationary observer. The equation that expresses this idea
is:
|
___________
Tm = |
__Tst___ |
|
(1-s2/c2)1/2 |
Tm = Time between the ticks of
the pointers of a moving clock
Tst = Time between the ticks
of the pointers of a stationary clock
Length and Mass
Just like time, the length and the mass of the object are also affected
by its velocity:
Lm =
Lst *
(1-s2/c2)1/2
Lm = Length of the object
moving at the speed s
Lst = Length of the same
object, when it is stationary
|
___________
Mm = |
__Mst___ |
|
(1-s2/c2)1/2 |
Mm = Mass of an object moving at
the speed s
Mst = Mass of the same object,
when it is stationary
In other words, the object is compressed and gets heavier when its speed
nears the speed of light.
Adding Velocities
The finite nature of the speed of light also implies that the addition of
high speeds doesn't work in the same way as the addition of velocities that are
common to us, because otherwise we would obtain superluminar velocities (higher
than the speed of light), that are forbidden by relativity. Being so:
|
____________
S = |
__s1 +
s2__ |
|
1+(s1*
v2)/c2 |
S = final speed
s1 = 1st added speed
s2 = 2nd added speed
If s1 and s2 are 10% of c, then:
|
____________
S = |
__0,1+0,1__ |
|
1+(0,1*0,1)/1 |
In other words, S = 0,2/1,01 = 0,198 (approximately 0,2 = 0,1 + 0,1)
If s1 and s2 are 90% of c, then:
|
____________
S = |
__0,9+0,9___ |
|
1+(0,9*0,9)/1 |
In other words, S = 1,8/1,81 = 0,994 (much lower than 1,8 = 0,9 + 0,9)
Gravity and Inertia
Relativity also tells us that the gravitational mass is equal to the inertial
mass (force that opposes the changes provoked in a massive body). Exemplifying,
a person that descends inside a lift falling under the influence of a
gravitational field feels like there isn't any gravity force acting on him. If
that person weighs himself on a balance located inside the lift, this will show
that he's weighing 0 kg, because the descending acceleration of the balance is
equal to the person's. It's like he weighed himself in the interplanetary
space, in the interior of a non-accelerated vehicle (where he would feel an
inertial force = 0).
In the same way, the effect felt by someone under the influence of a
gravitational field is equal to the one that is felt when he travels in a
vehicle far from any gravitational field but moving at an increasingly high
velocity - the person is pushed towards the inverse direction of the vehicle's
movement, as a car driver that suddenly steps on the brakes and feels pushed
against the front window. This is generally called the "principle of equivalence",
according to which the effects of a gravitational field can't be distinguished
from the effects of a uniform acceleration.
Consequences of the Principle of Equivalence
The immediate consequences of this principle are:
- The light beams are curbed under the
effect of a gravitational field, as in a lift accelerating in the space
(because the light follows a straight line when it's seen from the
outside but, given the acceleration of the lift, it follows a curved line
when it's seen from its interior). If in the presence of a gravitational
field a light beam is curbed, then the space-time (whose geometry is defined by
the course the light beams) is no longer flat, or in other words, the
pathway between 2 points is no longer a straight line. Given this,
gravitation determines the form of the space-time. Far away from the
source of the gravitational field, space-time becomes flat again. If the
mass of the object that creates the field is very small, then the
space-time around it also tends to be flat;
- An object, when it gets close to the
source of a gravitational field (for instance, a ball falling on the
floor), gains velocity as it descends and, this way, to its inertial
energy (E = Mc2) must be added the kinetic energy (speed). If
that energy is converted into a single photon (particle of light), then
this one will be more energetic when it reaches the floor, so its
frequency will be higher and, consequently, its wavelength will be
smaller (deviation to the blue). The opposite happens when a ball is
thrown to the air (or when a photon is sent to the opposite direction of
the source of the gravitational field). There is a deviation to the red.
Since a light wave with 1 mm of length is elongated, for instance, to 5
mm when it reaches a given height, then to the observer standing at the
top, the events occurring on the floor will look to be happening at a
slower pace because the time lapse between 2 light wave peaks (or
troughs) is higher than when this is observed from the floor. As the
accelerated reference vector is equivalent to a reference vector under
the effect of a gravitational, then the effect of the time dilation shall
be felt in a vehicle acceleratedly moving. So, the period between the ticks
of a clock placed inside this vehicle shall increase when that clock is
observed from the outside.
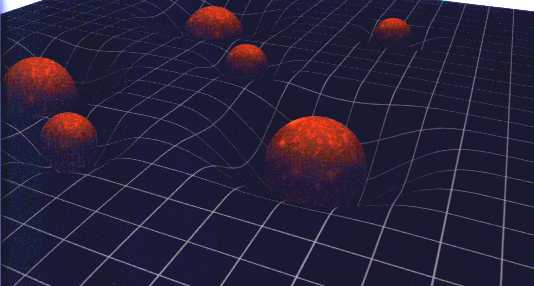
Curbed Space-Time (MoonRunner Design UK)

Clock at the base: 10:10
a.m., Clock at the top: 10 a.m. (MoonRunner Design UK)
Immediate
Consequences of the Theory of Relativity
The Twins Paradox
The most evident consequence of the theory of relativity is the
so-called "twins paradox", where is posed the hypothesis of a twin
(30 years old) that stays on the Earth while the other (also 30 years old)
departs to an interstellar trip at a velocity close to the light speed. At the
end of the trip, the one that stayed on the Earth (40 years old) should
acknowledge that his travelling twin would be younger (only 35 years old),
because his watch and his life functions would have been delayed by the
movement. However, the opposite should also succeed, since the twin brother
that stayed in the Earth moved in relation to the traveler twin (as movement is
relative and not absolute).
What actually happens is that the traveler twin comes back really
younger:
- The reference vector of the brother that
stayed on Earth is the inertial, since it wasn't subject to significant
velocity changes, besides the relatively modest movements over the
Earth's surface;
- The reference vector of the traveler twin
is not inertial, since the departure and the return imply that there had
to be at least one place where he changed the speed (between the time
when he was still going away and the time when he started to come back
again).
So, the age gap results from the use of 2 different reference vectors,
by each of the twin brothers. The accelerated reference vector is, as it was
mentioned, equivalent to a reference vector under the effect of a gravitational
field, which provokes the dilation of time. Therefore, when the accelerated
twin brother comes back, he shall be actually younger than his brother, just as
a twin brother that would have lived under the influence of a strong
gravitational field.
Other interesting consequences of relativity are the phenomena occurred
around a black hole. These objects are bodies where the mass is so concentrated
that, at its surface, the gravitational field is strong enough to prevent the
escape of the light itself, which moves at the maximum speed allowed by the
laws of physics. This hindrance is due to the fact that, under such conditions,
the course of the light is subject to an extreme bending, so high that it can
never be deviated from the centre of the gravitational field. As light isn't
able to escape from it, then nothing can be. The space-time (as the light) is disfigured in such a way that a
tunnel, called "wormhole", is formed. That tunnel will link the black
hole to a white hole (which expels matter, instead of swallowing it) placed in
a different universe or in a distant region of the same universe.

Black hole that is formed and gives origin to a
"wormhole" (MoonRunner Design UK)
In a rotating black hole, the tunnel takes the shape of a ring and not a
singularity. Opposing to what happens in a Schwarzchild black hole (without rotation),
it's possible to cross a Kerr black hole (with a rotation) at a speed lower
than that of the light. Nevertheless, the black holes with stellar origin exert
so strong tidal forces that they destroy everything that dares to enter in them
(as it happens to a massive body in the interior of the Roche limit). A super-massive black hole, as the one that
possibly exists in the heart of our galaxy, doesn't pose so many problems
concerning to this issue, because its surface (the events horizon) is too far away from the centre of the gravitational
field. Obviously this kind of black holes is very seldom, since they occur only
in very special locations (it is thought that only
one exists in the Milky Way). Whoever may cross it will have, though, the
chance to reach another universe or a distant place in our universe.
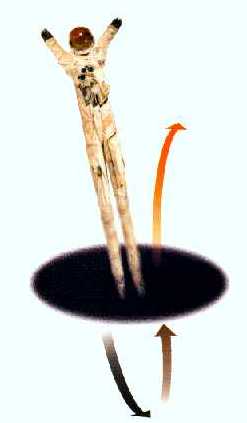
Tidal forces on the
surface of a stellar black hole (MoonRunner Design UK)
Backward Time Travel
The existence of wormholes suggests that it is theoretically possible
for someone to effectuate backward travels in time. The wormholes are
comparable to shortcuts in the geometry of space-time, or in other words, they
allow the connection between 2 different points of one or two universes, with
local times that can be distinct.
Let's suppose that one wormhole is linking Earth to Alpha Centauri (the
closest star apart from the Sun). If one of the mouths of the wormhole is kept
stationary while the other is accelerated and braked further, then this one, as
the travelling twin, will be younger that the mouth that didn't move.
Let's imagine that the mouth close to the Earth is now 6 years younger
than the mouth in Alpha Centauri. If we travel from the Earth to out neighbouring
star in 5 years, get into the tunnel through the mouth that is located there
and get out of the tunnel through the mouth located at the Earth of 6 years
ago, then we will have arrived to the starting point one year before we left.
The Paradoxes of Time Travels
This kind of travels in time generates a paradox: what happens if I
begin a trip until a time when my father was not born yet and, when I arrive
there, I decide to kill my paternal grandfather? Without my grandfather I could
have never be born, so I wouldn't be able to make the trip. This way, my
grandfather would be kept alive and I, after all, would have been born.
There are 2 proposed solutions to this paradox:
- The approach of the coherent stories
supports that even if the trip to the past is possible, whatever happens
in the space-time shall agree with the laws of the physics. According to
this theory, when I would begin the time travel I would already have the
memory of all the consequences of that trip and wouldn't be able to
change, in any way, the course of the events. That implies that the
traveler won't have neither freedom of choice nor free will;
- The approach of the alternative stories
supports that when the traveler makes the trip he enters in an
alternative story, different from the memorized story. He would then be
free to act with freedom, not constrained. This approach seems to agree
with the Feynman's quantum
theory of the sum of the stories, which advocates that there
is an infinity of possible stories, each one with a determined
probability.
-